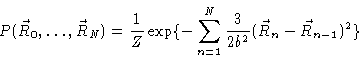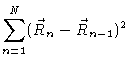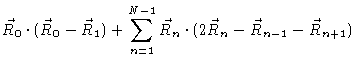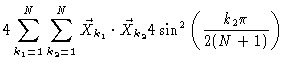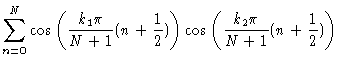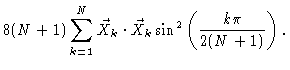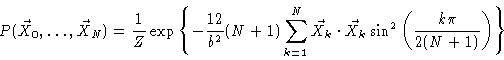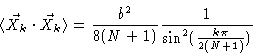Appendix A

In this appendix we calculate the equilibrium expectation value of X_k². In Cartesian coordinates the statistical weight of some configuration 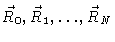$\vec{R }_0,\vec{R}_1,\ldots,\vec{R}_N$ is given by

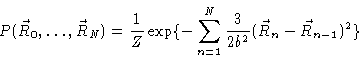$$P(\vec{R}_0,\ldots,\vec{R}_N) = \frac{1}{Z} \exp \{ -\sum_{n=1}^N \frac{3}{ 2b^2} (\vec{R}_n-\vec{R}_{n-1})^2 \}$$ (6.77)

Since the transformation to normal coordinates is a linear transformation from one set of orthogonal coordinates to another, the corresponding Jacobian is simply a constant. The argument of the exponential may be written as

$${\sum_{n=1}^N (\vec{R}_n - \vec{R}_{n-1})^2 }$$

$$\vec{R}_0 \cdot (\vec{R}_0 - \vec{R}_1) + \sum_{n=1}^{N-1} \vec{R}_n \cdot (2\vec{R}_n - \vec{R}_{n-1} - \vec{R}_{n+1})$$ =

$$+ \vec{R}_N \cdot (\vec{R}_N - \vec{R}_{N-1})$$

$$4 \sum_{k_1=1}^N \sum_{k_2=1}^N \vec{X}_{k_1} \cdot \vec{X}_{k_2} 4 \sin^2 \left( \frac{k_2\pi}{2(N+1)} \right)$$ =

$$\sum_{n=0}^N \cos \left( \frac{k_1\pi}{N+1} (n+\frac{1}{2} ) \right) \cos \left( \frac{k_2\pi}{N+1} (n+\frac{1}{2}) \right)$$

$$8(N+1) \sum_{k=1}^N \vec{X}_k \cdot \vec{X}_k \sin^2 \left( \frac{k\pi }{2(N+1)} \right) .$$ = (6.78)

The statistical weight therefore reads

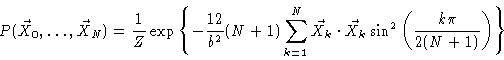$$P(\vec{X}_0,\ldots,\vec{X}_N) = \frac{1}{Z} \exp \left\{ - \f... ... \vec{X}_k \sin^2 \left( \frac{k\pi}{2(N+1) } \right) \right\}$$ (6.79)

Since this is a simple product of independent Gaussians, all kinds of expectation values may easily be calculated, like for example

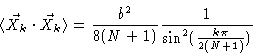$$\langle \vec{X}_k \cdot \vec{X}_k \rangle = \frac{b^2}{8(N+1)} \frac{1}{ \sin^2 (\frac{k\pi}{2(N+1)}) }$$ (6.80)